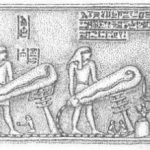
Despite their superstitions, Egyptian priests encouraged the development of many scientific disciplines, especially astronomy and mathematics. The construction of the pyramids and other astonishing monuments would have been impossible without a highly developed mathematical knowledge. The Rhind Mathematical Papyrus (also known as the Ahmes Papyrus) is an ancient mathematical treatise, dating back to approximately 1650 BCE. This work explains, using several examples, how to calculate the area of a field, the capacity of a barn, and it also deals with algebraic equations of the first degree. In the opening section, its author, a scribe named Ahmes, declares that the Papyrus is a transcription of an ancient copy, possibly 500 years before the time of Ahmes himself.
The flooding of the Nile, which constantly altered the border markers that separated the different portions of land, also encouraged the development of mathematics: Egyptian land surveyors had to perform measurements over and over again to restore the boundaries that had been lost. In fact, this is the origin of the word geometry: “measurement of land”. Egyptian land surveyors were very practical minded: in order to form right angles, which was critical for establishing the borders of a field, they used a rope divided into twelve equal parts, forming a triangle with three parts on one side, four parts on the second side, and five parts on the remaining side. The right angle was to be found where the three-unit side joined the four-unit side. In other words, Egyptians knew that a triangle whose sides are in a 3:4:5 ratio is a right triangle. This is a useful rule of thumb and it is also a step away from the Pythagoras Theorem, which is based on stretching the 3:4:5 triangle concept to its logical limit.
Egyptians calculated the value of the mathematical constant pi at 256/81 (3.16), and for the value of the square root of two, they used the fraction 7/5 (which they thought of as 1/5 seven times). For fractions, they always used the numerator 1 (in order to express 3/4, they wrote 1/2 + 1/4). Unfortunately they did not know the zero, and their numeral system lacked simplicity: 27 signs were required to express 999.

Greek Science
Unlike other parts of the world were science was strongly connected with religion, Greek scientific thought had a stronger connection with philosophy. As a result, the Greek scientific spirit had a more secular approach and was able to replace the notion of supernatural explanation with the concept of a universe that is governed by laws of nature. Greek tradition credits Thales of Miletus as the first Greek who, around 600 BCE, developed the idea that the world can be explained in natural terms. Thales lived in Miletus, a Greek city locate in Ionia, the central sector of Anatolia’s Aegean shore in Asia Minor, present-day Turkey. This city was the main focus of the “Ionian awakening”, the initial phase of classical Greek civilization, a time when the ancient Greeks developed a number of ideas surprisingly similar to some of our modern scientific concepts.
One of the great advantages of Greece was the influence of Egyptian mathematics, when Egypt opened its ports to Greek trade during the 26th Dynasty (c. 685–525 BCE) and Babylonian astronomy, after Alexander’s conquest of Asia Minor and Mesopotamia during Hellenistic times. The Greeks were very talented at systematically innovating upon the Egyptian and Babylonian mathematical and astronomical knowledge. This turned the Greeks into some of the most competent mathematicians and astronomers of antiquity and their achievements in geometry were arguably the finest.
While observation was important at the beginning, Greek science eventually began to undervalue observation in favour of the deductive process, where knowledge is built by means of pure thought. This method is key in mathematics and the Greeks put such an emphasis on it that they falsely believed that deduction was the way to obtain the highest knowledge. Observation was underestimated, deduction was made king, and Greek scientific knowledge was led up a blind alley in virtually every branch of science other than exact sciences (mathematics).